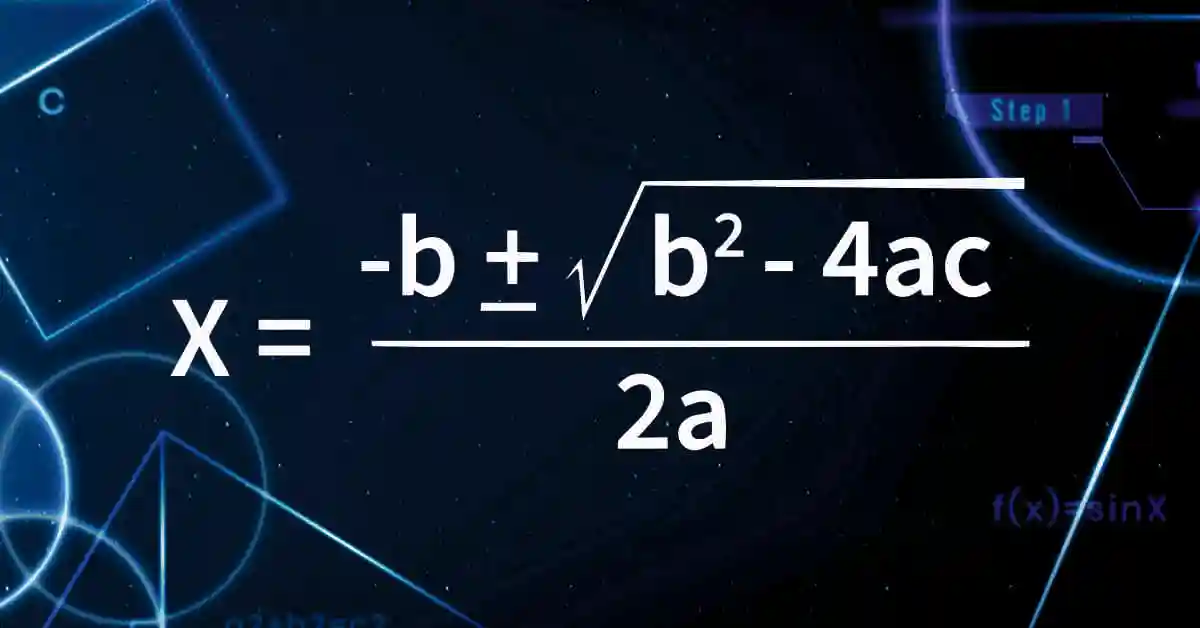What is the Quadratic formula?
In mathematics, the quadratic formula is a highly effective technique for solving quadratic equations and finding their roots.
The formula for calculating the roots of a quadratic equation
ax2 + bx + c = 0 is given by x = [-b ± √(b2 – 4ac)]/2a
Here, D=b2−4acD = b^2 – 4acD=b2−4ac is known as the discriminant of the quadratic equation. The value of the discriminant determines the nature of the roots of the quadratic equation.
- If D < 0, the roots are not real, i.e., imaginary.
- If D = 0, the roots are real and equal.
- If D > 0, the roots are real and unequal.
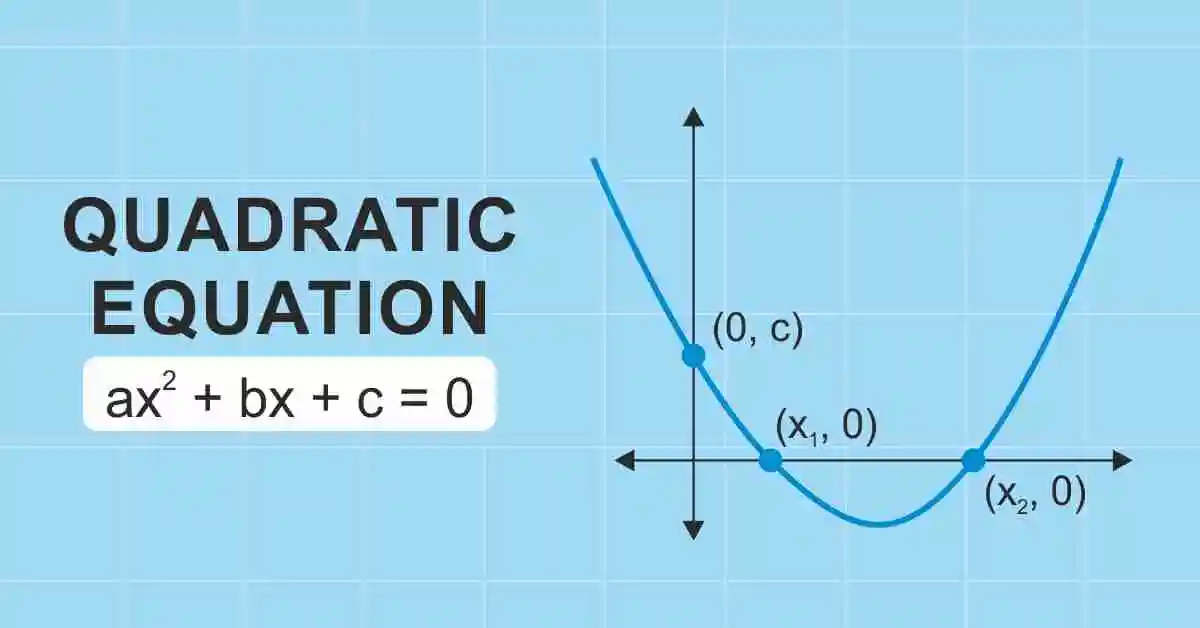We have compiled Quadratic formula questions to assist students in Classes 10 and 11 with solving quadratic equations, particularly those with imaginary roots.
While the factorization technique is often straightforward for finding the roots of quadratic equations, it may not always be applicable. In such instances, the quadratic formula becomes an essential tool, enabling us to find the roots of quadratic equations effortlessly.
Recommended reading: The Best Color by Number Books for Kids
However, the quadratic formula is used to find the roots of a quadratic equation when other methods are not sufficient, particularly for finding imaginary roots. Let’s delve into a detailed discussion about the quadratic formula and its derivation here.
Problems with Solution:
m2 − 31 − 2m = −6 − 3m2 − 4m
Given,
m2 − 31 − 2m = −6 − 3m2 − 4m
m2 – 31 – 2m + 6 + 3m2 + 4m = 0
4m2 + 2m – 25 = 0
Comparing with the standard form of quadratic equation ax2 + bx + c = 0, we get;
a = 4, b = 2 and c = -25
Now, b2 – 4ac = (2)2 – 4(4)(-25)
= 4 + 400
= 404 > 0
That means the given equation has two real and unequal roots.
Using the quadratic formula,
x = [-b ± √(b2 – 4ac)]/2a
m = [-2 ± √404]/ 2(4)
= [-2 ± √(4 × 101)]/8
= (-2 ± 2√101)/8
Therefore, m = (-2 + 2√101)/8 and m = (-2 – 2√101)/8.
Write the roots of the quadratic equation 3×2 +3x = -3 using the quadratic formula.
Solution:Given,
3×2 +3x = -3
3×2 +3x + 3 = 0
3(x2 + x + 1) = 0
x2 + x + 1 = 0
Here, a = 1, b = 1 and c = 1.
Using the quadratic formula,
x = [-b ± √(b2 – 4ac)]/2a
= [-1 ± √{12 – 4(1)(1)}]/ 2(1)
= [-1 ± √(1 – 4)]/ 2
= (-1 ± √-3)/2
= (-1 ± i√3)/2
x = (-1 + i√3)/2, x = (-1 – i√3)/2
Therefore, the roots of 3×2 + 3x = -3 are (-1 + i√3)/2 and (-1 – i√3)/2
Using the quadratic formula, find the roots of the quadratic equation 2×2 – 7x + 6 = 0.
Given,
2×2 – 7x + 6 = 0
When compared with the standard form, we have;
a = 2, b = -7 and c = 6
D = b2 – 4ac
= (-7)2 – 4(2)(6)
= 49 – 48
= 1 > 0
That means the roots are real and unequal.
Using the quadratic formula,
x = [-b ± √(b2 – 4ac)]/2a
= [-(-7) ± √1]/ 2(2)
= (7 ± 1)/4
x = (7 + 1)/4, x = (7 – 1)/4
x = 8/4, x = 6/4
x = 2, x = 3/2
Therefore, 2 and 3/2 are the roots of the given equation.
Find whether the equation 5×2 – 2x – 10 = 0 has real roots. If real roots exist, find them.
Given,
5×2 – 2x – 10 = 0
Here, a = 5, b = -2, and c = -10
Let us calculate the discriminant value.
D = b2 – 4ac
= (-2)2 – 4(5)(-10)
= 4 + 200
= 204 > 0
That means the given quadratic equation has two real and unequal roots.
Now, using the quadratic formula, we have;
x = [-b ± √(b2 – 4ac)]/2a
= [-(-2) ± √204]/ 2(5)
= (2 ± √(4 × 51))/10
x = (2 + 2√51)/10, x = (2 – 2√51)/10
x = 2(1 + √51)/10, x = 2(1 – √51)/10
x = (1 + √51)/5 , x = (1 – √51)/5
Therefore, the real roots of the given quadratic equation are (1 + √51)/5 and (1 – √51)/5.
Calculate the roots of the quadratic equation x – 1/x = 3, x ≠ 0, using the quadratic formula.
Given the quadratic equation is:
x – 1/x = 3
(x2 – 1)/x = 3
x2 – 1 = 3x
x2 – 3x – 1 = 0
Here, a = 1, b = -3 and c = -1
D = b2 – 4ac
= (-3)2 – 4(1)(-1)
= 9 + 4
= 13 > 0
Thus, the roots of the given quadratic equation are real and unequal.
Using the quadratic formula,
x = [-b ± √(b2 – 4ac)]/2a
= [-(-3) ± √13]/ 2(1)
= (3 ± √13)/2
x = (3 + √13)/2, x = (3 – √13)/2
Hence, (3 + √13)/2 and (3 – √13)/2 are the roots of the given quadratic equation.
What are the roots of the quadratic equation 2×2 – √5x + 1 = 0?
Given,
2×2 – √5x + 1 = 0
Here, a = 2, b = -√5 and c = 1
D = b2 – 4ac
= (-√5)2 – 4(2)(1)
= 5 – 8
= -3 < 0
That means the roots are imaginary.
Using the quadratic formula, we have;
x = [-b ± √(b2 – 4ac)]/2a
= [-(-√5) ± √(-3)]/ 2(2)
= (√5 ± i√3)/4
x = (√5 + i√3)/4, (√5 – i√3)/4
Hence, (√5 + i√3)/4 and (√5 – i√3)/4 are the roots of the quadratic equation 2×2 – √5x + 1 = 0.
Find the roots of the quadratic equation 4×2 + 4√3x + 3 = 0
Given,
4×2 + 4√3x + 3 = 0
Here, a = 4, b = 4√3 and c = 3
b2 – 4ac = (4√3)2 – 4(4)(3)
= 48 – 48
= 0
That means the roots of the given quadratic equation are real and equal.
By using the quadratic formula, we get,
x = [-b ± √(b2 – 4ac)]/2a
x = (-4√3 ± √0)/8
x = (-4√3)/8
x = -√3/2
Therefore, -√3/2 and -√3/2 are the roots of the given equation.
What will be the roots of the quadratic equation 10×2 − 9x + 6 = 0?
Given the quadratic equation is:
10×2 − 9x + 6 = 0
Here, a = 10, b = -9 and c = 6.
b2 – 4ac = (-9)2 – 4(10)(6)
= 81 – 240
= -159
Using the quadratic formula, we have;
x = [-(-9) ± √(b2 – 4ac)]/2a
= [9 ± √(-159)]/ 2(10)
= (9 ± i√159)/20
x = (9 + i√159)/20, (9 – i√159)/20
Hence, (9 + i√159)/20 and (9 – i√159)/20 are the roots of the quadratic equation 10×2 − 9x + 6 = 0.
Solve the following equation using the quadratic formula:4×2 – 1 = -8x
Given,
4×2 – 1 = -8x
4×2 + 8x – 1 = 0
Here, a = 4, b = 8 and c = -1
Now, b2 – 4ac = (8)2 – 4(4)(-1)
= 64 + 16
= 80 > 0
That means the given quadratic equation contains two real and unequal roots.
Now, by using the quadratic formula, we have;
x = [-b ± √(b2 – 4ac)]/2a
= [-8 ± √80]/ 2(4)
= (-8 ± √(16 × 5))/8
x = (-8 + 4√5)/8, x = (-8 – 4√5)/8
x = 4(-2 + √5)/8, x = 4(-2 – √5)/8
x = (-2 + √5)/2, x = (-2 – √5)/2
Therefore, the real roots of the given quadratic equation are (-2 + √5)/2 and x = (-2 – √5)/2.
What is the nature of the roots of 5×2 + 3x + 1 = 0? Find them.
Given,
5×2 + 3x + 1 = 0
Here, a = 5, b = 3 and c = 1.
Let us calculate the discriminant to determine the nature of the roots of 5×2 + 3x + 1 = 0.
D = b2 – 4ac
= (3)2 – 4(5)(1)
= 9 – 20
= -11 < 0
Thus, the given quadratic equation contains two imaginary roots.
Using the quadratic formula, we have;
x = [-b ± √(b2 – 4ac)]/2a
= [-3 ± √(-11)]/ 2(5)
= (-3 ± i√11)/10
x = (-3 + i√11)/10, (-3 – i√11)/10
Hence, (-3 + i√11)/10 and (-3 – i√11)/10 are the roots of the given quadratic equation.
Solve the quadratic equation using the quadratic formula. 2𝑥² −𝑥=1
2 x² –x–1=0
𝑎= 2
𝑏= –1
𝑐= –1
𝑥= –𝑏± √𝑏² –4𝑎𝑐/2𝑎
= – (–1) ±√(–1)² –4(2) (–1)/2(2)
=1±√1+8/4
=1±√9/4
=1±3/4
X1=1+3/4
=4/4
=1
X2=1-3/4
= –2/4
= –1/2
Therefore, the answers are 𝑥1=1 𝑥2= –1/2
Conclusion
Practicing these problems will boost your confidence in using the quadratic formula to solve any quadratic equation. Remember, you have to carefully follow each step and double-check your calculation. With regular practice, you’ll find solving quadratic equations using the quadratic formula to be straightforward and efficient.
Want to excite your child about math and sharpen their math skills? Moonpreneur’s online math curriculum is unique as it helps children understand math skills through hands-on lessons, assists them in building real-life applications, and excites them to learn math.
You can opt for our Advanced Math or Vedic Math+Mental Math courses. Our Math Quiz for grades 3rd, 4th, 5th, and 6th helps in further exciting and engaging in mathematics with hands-on lessons.
Recommended reading: The International Mathematical Olympiad
