Triangles are fundamental geometric shapes, forming the building blocks for more complex polygons and shapes. One of the critical aspects of a triangle is its vertices – those corner points where the sides of the triangle meet.
Understanding triangle vertices is crucial for basic geometry, advanced mathematics, and applications in various fields like computer graphics, physics, and engineering. This blog will delve into triangle vertices with examples to deepen our understanding.
What are Triangle Vertices?
Vertices (singular: vertex) are the points where the sides of a triangle intersect. Every triangle has three vertices, and these points collectively define the shape and properties of the triangle.
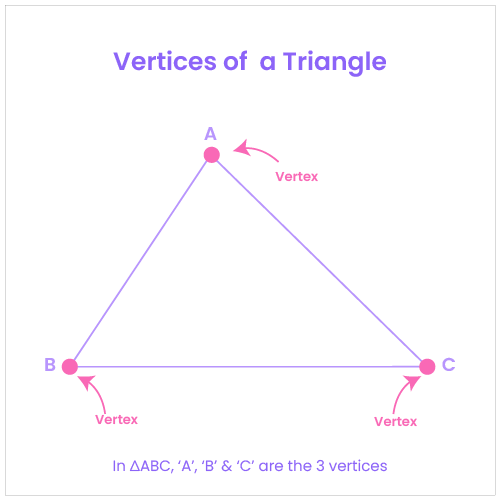
The vertices are typically named using capital letters A, B, and C.
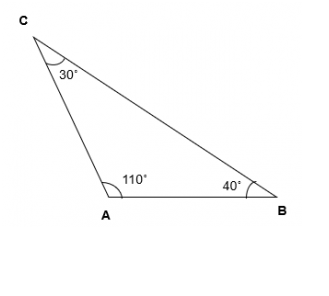
Example 1:
Consider the following triangle:
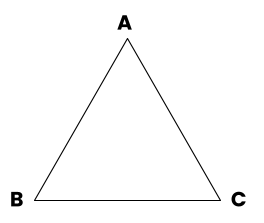
In this triangle, the vertices are labeled A, B, and C. The sides are AB, BC, and CA.
Properties and Characteristics
Understanding the vertices of a triangle opens up a world of insights into the triangle’s properties and characteristics.
Triangle Types Based on Angles:
Acute Triangle: All angles are less than 90 degrees.
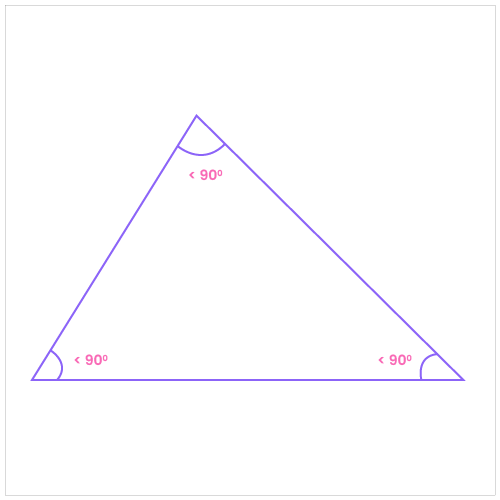
Obtuse Triangle: One angle is greater than 90 degrees.
Right Triangle: One angle is precisely 90 degrees.
Triangle Types Based on Sides:
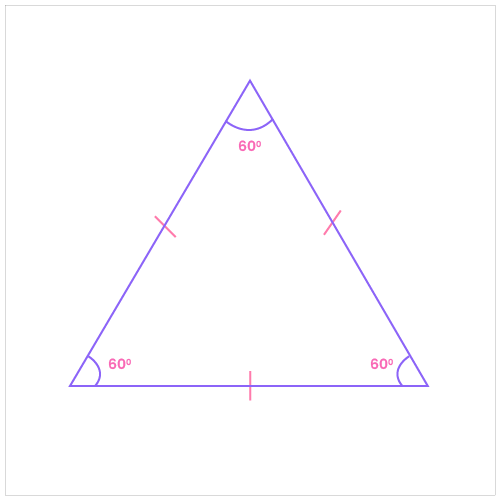
Equilateral Triangle: All sides are of equal length.
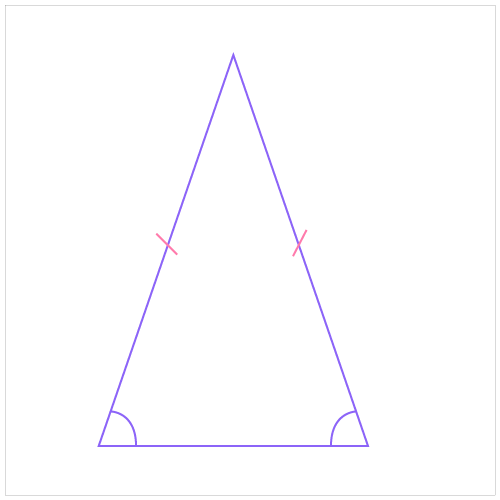
Isosceles Triangle: Two sides are of equal length.
Scalene Triangle: All sides have different lengths.
Angle Sum Property:
The sum of the angles in any triangle always equals 180 degrees. This property is a consequence of a straight line measuring 180 degrees.
Example 2:
Consider a triangle with angle measurements: ∠A = 50°, ∠B = 70°, and ∠C = 60°.
Using the angle sum property, we can verify that:
50° + 70° + 60° = 180°
Thus, the angles satisfy the angle sum property, confirming that they form a triangle.
Recommended Reading: Understanding Axis of Symmetry
Coordinate Geometry and Triangle Vertices
Triangle vertices can also be represented using coordinate geometry. If you know the coordinates of the three vertices, you can determine various properties of the triangle, such as its area, perimeter, and orientation.
Example 3:
Given the vertices A(2, 3), B(5, 7), and C(8, 2), you can calculate the lengths of the sides AB, BC, and CA using the distance formula.
Furthermore, you can find the area of the triangle using methods like the Shoelace Formula or by treating it as half the area of a parallelogram formed by the vectors AB and AC.
Real-World Applications
Understanding triangle vertices is essential in various fields:
Computer Graphics
Vertices play a crucial role in defining the geometry of shapes in 3D computer graphics.
Engineering
We use Triangles in finite element analysis to model complex structures.
Physics
In physics simulations, triangles can represent facets of objects, aiding in simulations of natural phenomena.
Navigation
Triangles formed by landmarks and positions are used in trigonometric methods for navigation and surveying.
Conclusion
Triangle vertices are not just points on a shape; they unlock a wealth of geometric and mathematical knowledge.
From defining basic triangle types to enabling advanced calculations in various fields, vertices play a vital role. By understanding triangle vertices, we gain insight into the intricate world of geometry and its practical applications.
Moonpreneur understands the needs and demands this rapidly changing technological world is bringing with it for our kids. Our expert-designed Advanced Math course for grades 3rd, 4th, 5th, and 6th will help your child develop math skills with hands-on lessons, excite them to learn, and help them build real-life applications.
Register for a free 60-minute Advanced Math Workshop today!
