The Order of Operations in Math — A Kid-Friendly Guide
Understanding the order of operations is very important in mathematics if you have to solve equations correctly. Without these, calculations could get complex and lead to getting the wrong answer. Perhaps you have an exam tomorrow or next week, and you wish to refresh your understanding, then this comprehensive guide will aid you in masterfully perfecting the art of operations with practical examples, definitions, and questions and answers.
What is the Order of Operations?

Have you ever tried to solve a math problem but didn’t know where to start? For instance, when you look at 3 + 8 × 2 – 6, what do you do first?
- Add 3 and 8?
- Multiply 8 and 2?
- Subtract 6 from 2?
The order of operations is the rules which tell us where to start in a mathematical expression containing more than one operation such as addition, subtraction, multiplication, or division. The correct outcome will always be achieved following the order.
Example:
Let’s solve 3 + 8 × 2 – 6 using the correct steps:
- Perform multiplication: 8 × 2 = 16
- Add: 3 + 16 = 19
- Subtract: 19 – 6 = 13
The right answer is 13.
We remember how to work it out by using an acronym, PEMDAS:
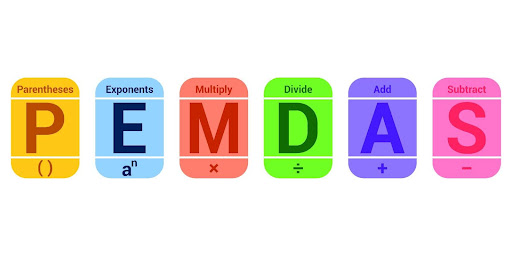
P: Parentheses solve the expression inside first
E: Exponents (solve powers or square roots)
MD: Multiplication and Division, solve from left to right
AS: Addition and Subtraction, solve from left to right
There are some other versions of the mnemonic in different countries: BODMAS (Brackets, Orders, Division, Multiplication, Addition, Subtraction) and BEDMAS (Brackets, Exponents, Division, Multiplication, Addition, Subtraction).
Steps in PEMDAS with Examples
1. Parentheses
Always evaluate what’s in the parentheses first.
Example: 3 × (4 + 2)
- Evaluates what’s inside the parentheses: 4 + 2 = 6
- Multiply: 3 × 6 = 18
Sometimes, expressions contain multiple layers of parentheses:
Example: {4 × [5 + (6 – 3)]} – (8 – 2)
- First, evaluate the innermost parentheses: 6 – 3 = 3 → {4 × [5 + 3]} – (8 – 2)
- Next layer to solve: 5 + 3 = 8 → {4 × 8} – (8 – 2)
- Multiply: 4 × 8 = 32 → 32 – (8 – 2)
- Next to Solve the parentheses: 8 – 2 = 6 → 32 – 6
- Subtract: 32 – 6 = 26
2. Exponents
Exponents are how many times a number is multiplied by itself.
Example: 3² + 4 × 2
- Solve the exponent: 3² = 9 → 9 + 4 × 2
- Multiply: 4 × 2 = 8 → 9 + 8
- Add: 9 + 8 = 17
3. Multiply and Division
Do all from left to right.
Example: 8 ÷ 2 × 4 + 3
- Divide: 8 ÷ 2 = 4 → 4 × 4 + 3
- Multiply: 4 × 4 = 16 → 16 + 3
- Add: 16 + 3 = 19
4. Add and Subtract
Perform these from left to right.
Example: 10 – 4 + 3
- Subtract: 10 – 4 = 6 → 6 + 3
- Add: 6 + 3 = 9
Common Mistakes Using the Order of Operations Math
Understanding the order of operations is critical to get the correct solutions for math equations. Students, however, are often victims of common pitfalls while working with PEMDAS: Parentheses, Exponents, Multiplication and Division, Addition and Subtraction.
Let’s look at some typical errors and how to not make them:
1. Missing the Parentheses
Parentheses should always be dealt with first, but many students miss this and end up with an incorrect answer.
Example:
Solve 5×(2+6)−45 \times (2 + 6) – 45×(2+6)−4.
Mistake: Doing 5×2=105 \times 2 = 105×2=10, then 10+6−4=1210 + 6 – 4 = 1210+6−4=12.
Correct: Begin with the parentheses:
5×(2+6)−4=5×8−4=40−4=36.5 \times (2 + 6) – 4 = 5 \times 8 – 4 = 40 – 4 = 36. 5×(2+6)−4=5×8−4=40−4=36.
2. Treating Multiplication as Always First
Most believe that multiplication is always to be done before division, but both are equal and must be done from left to right.
Practice:
Solve 24÷4×324 \div 4 \times 324÷4×3.
Mistake: Doing 4×3=124 \times 3 = 124×3=12, then 24÷12=224 \div 12 = 224÷12=2.
Correct Procedure: Do left to right:
24÷4×3=6×3=18.24 \div 4 \times 3 = 6 \times 3 = 18. 24÷4×3=6×3=18.
3. Misapplication of Addition and Subtraction
Addition and subtraction are equally weighted. If they are calculated out of order, incorrect solutions are obtained.
Example:
Solve 15−5+1015 – 5 + 1015−5+10.
Mistake: Adding first: 5+10=155 + 10 = 155+10=15, then 15−15=015 – 15 = 015−15=0.
Correct Approach: Work left to right:
15−5+10=10+10=20.15 – 5 + 10 = 10 + 10 = 20. 15−5+10=10+10=20.
4. Failure to consider Exponents
Exponents are often overlooked or incorrectly calculated, changing the entire outcome.
Example:
Solve 2×32−42 \times 3^2 – 42×32−4.
Mistake: Multiplying first: 2×3=62 \times 3 = 62×3=6, then 62−4=36−4=326^2 – 4 = 36 – 4 = 3262−4=36−4=32.
Correct Approach: Apply the exponent first:
2×32−4=2×9−4=18−4=14.2 \times 3^2 – 4 = 2 \times 9 – 4 = 18 – 4 = 14. 2×32−4=2×9−4=18−4=14.
Examples Using PEMDAS
Example 1:
Solve 4+(5×2)2÷104 + (5 \times 2)^2 \div 104+(5×2)2÷10.
- Parentheses: 5×2=105 \times 2 = 105×2=10.
- Exponent: 102=10010^2 = 100102=100.
- Division: 100÷10=10100 \div 10 = 10100÷10=10.
- Addition: 4+10=144 + 10 = 144+10=14.
Answer: 14
Example 2:
Solve (12−8)×3+23÷4(12 – 8) \times 3 + 2^3 \div 4(12−8)×3+23÷4.
- Parentheses: 12−8=412 – 8 = 412−8=4.
- Exponent: 23=82^3 = 823=8.
- Multiplication: 4×3=124 \times 3 = 124×3=12.
- Division: 8÷4=28 \div 4 = 28÷4=2.
- Addition: 12+2=1412 + 2 = 1412+2=14.
Answer: 14
Example 3:
Solve 60÷(3+2)×4−760 \div (3 + 2) \times 4 – 760÷(3+2)×4−7.
- Parentheses: 3+2=53 + 2 = 53+2=5.
- Division: 60÷5=1260 \div 5 = 1260÷5=12.
- Multiplication: 12×4=4812 \times 4 = 4812×4=48.
- Subtraction: 48−7=4148 – 7 = 4148−7=41.
Answer: 41
Conclusion
Understanding order of operations in maths is akin to learning to play a game. Having understood the right steps–Parentheses, Exponents, Multiplication and Division (from left to right), and Addition and Subtraction (from left to right)-math problems can now be solved accurately and also timely. One can not forget to use the name given to the steps itself –PEMDAS. With practice, you will be confident and ready to face even the toughest math problems! So, keep practicing and let the order of operations guide you to success!
You can opt for our Advanced Math or Vedic Math+Mental Math courses. Our Math Quiz for grades 3rd, 4th, 5th, and 6th helps in further exciting and engaging in mathematics with hands-on lessons.
Frequently Asked Questions About PEMDAS
Always work on the innermost parentheses first and gradually solve outward.
Example:
Solve 6÷[3+(2×4)]6 \div [3 + (2 \times 4)]6÷[3+(2×4)].
- Innermost Parentheses: 2×4=82 \times 4 = 82×4=8.
- Outer Parentheses: 3+8=113 + 8 = 113+8=11.
- Division: 6÷11≈0.556 \div 11 \approx 0.556÷11≈0.55.
They are variations of the same rule.
BODMAS: Brackets, Orders, Division/Multiplication, Addition/Subtraction.
PEMDAS: Parentheses, Exponents, Multiplication/Division, Addition/Subtraction.
Solve the innermost parentheses first and move outward.
Example:
Solve [(5+3)×2]+(6÷3)[(5 + 3) \times 2] + (6 \div 3)[(5+3)×2]+(6÷3):
- Innermost: 5+3=85 + 3 = 85+3=8, 6÷3=26 \div 3 = 26÷3=2.
- Next: 8×2=168 \times 2 = 168×2=16.
- Final: 16+2=1816 + 2 = 1816+2=18.
Yes, multiplication and division take precedence over addition and subtraction. However, if multiplication/division or addition/subtraction appear together, solve them from left to right.
Substitute the values of the variables first, then follow PEMDAS.
Example:
Solve 3x+2y3x + 2y3x+2y when x=4x = 4x=4 and y=5y = 5y=5:
- Substitute: 3(4)+2(5)3(4) + 2(5)3(4)+2(5).
- Multiply: 12+1012 + 1012+10.
- Add: 222222.
PEMDAS applies to fractions. Simplify the numerator and denominator separately before dividing.
Example:
Simplify (6+4)÷(8−2)(6 + 4) \div (8 – 2)(6+4)÷(8−2):
- Numerator: 6+4=106 + 4 = 106+4=10.
- Denominator: 8−2=68 – 2 = 68−2=6.
- Divide: 10÷6=5/310 \div 6 = 5/310÷6=5/3
The difference is in terminology. GEMDAS replaces “Parentheses” with “Grouping Symbols,” which encompasses a broader range of symbols such as brackets, braces, and fraction bars.
Treat the fraction bar as a grouping symbol. Solve the numerator and denominator separately, then divide.
Example:
Simplify 8+24×2\frac{8 + 2}{4 \times 2}4×28+2:
- Numerator: 8+2=108 + 2 = 108+2=10.
- Denominator: 4×2=84 \times 2 = 84×2=8.
- Fraction: 10/8=5/410/8 = 5/410/8=5/4.
Exponents follow parentheses, which are the highest priority, and come ahead of all other operations multiplication, division, addition, or subtraction. Simplify any exponents before moving to the operations.
Example:
Simplify 3+233 + 2^33+23:
- Exponent: 23=82^3 = 823=8.
- Add: 3+8=113 + 8 = 113+8=11.
Begin with the innermost exponent and work outwards
Example:
Solve 2322^{3^2}232:
- Simplify the inner exponent: 32=93^2 = 932=9.
- Calculate the outer exponent: 29=5122^9 = 51229=512.

















Learning the order of operations is so important, especially as kids move to more complex math problems. It’d be great to pair this concept with real-world scenarios, like budgeting or recipe measurements, to show how math applies in everyday life!
I still remember when my daughter first learned the order of operations—she used to get so confused with the parentheses and exponents! Once we started using fun examples and breaking it down step by step, it clicked for her. Now, she’s solving equations faster than me!