Update: This article was last updated on 15th July 2025 to reflect the accuracy and up-to-date information on the page.
Cracking the Code: An Introduction to the Quadratic Formula
Have you ever run across an equation hiding a variable like x under a square? if so, then you know what a quadratic equation is! And, while they look slightly scary at first, quadratic equations can still be solved using a powerful tool known as the quadratic formula. This blog will introduce you to quadratic equations and how to solve them using the quadratic formula.
Unveiling the Quadratic Beast
A quadratic equation is an algebraic equation in which the highest power of the unknown variable (typically x) is 2. This means that it has terms such as x^2 (x squared), and possibly x (x to the first power), and a constant. Here is the general form of a quadratic equation:
ax^2 + bx + c = 0
Where a, b, and c are constants, and a ≠ 0 (otherwise it wouldn’t be a quadratic equation!). The end goal is to find x (the solutions) so that the equation is satisfied.
There are a few ways to solve quadratic equations. Occasionally, you might be lucky enough to factor the left side of the equation easily, paving the way to the solutions. However, what if you can’t? This is where the quadratic formula comes in as a universally applicable solution to every quadratic equation.
Recommended Reading: Quadratic formula song: A fun way to learn quadratic equations.
The Quadratic Formula: A Universal Decoder
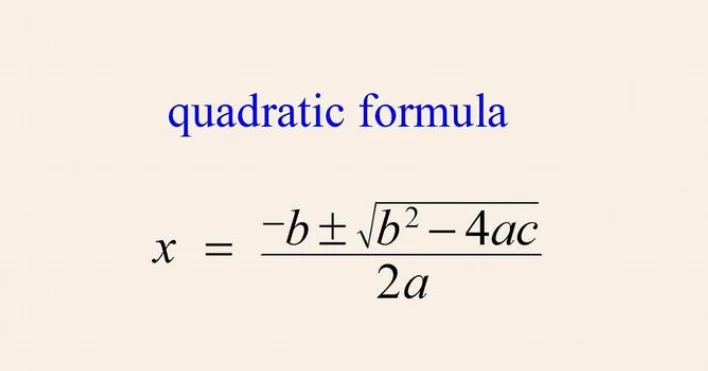
The quadratic formula is a mathematical equation that provides the solutions for any quadratic equation in the form ax^2 + bx + c = 0. Here it is in all its glory:
x = (-b ± √(b^2 – 4ac)) / (2a)
Don’t be intimidated by the symbols! Let’s break down what this formula means:
- x: This represents the unknown variable you’re solving for.
- a, b, and c: These are the constants from your original quadratic equation (ax^2 + bx + c = 0).
- ±: This symbol represents “plus or minus.” The formula gives you two possible solutions, so you’ll need to evaluate both sides of the equation separately to find them.
- √: This symbol represents the square root.
- b^2 – 4ac: This part of the formula is called the discriminant. It determines the nature of the solutions we’ll get. We’ll delve into this later.
Recommended Reading: A deep dive into the meaning of tangents in math
Using the Formula: Step-by-Step
Now that you know the formula, let’s see it in action! Here’s a sample quadratic formula equation:
2x^2 + 5x – 3 = 0
We want to find the solutions (values of x) that satisfy this equation. Here’s how to use the formula:
- Identify the values of a, b, and c: In this case, a = 2, b = 5, and c = -3.
- Plug the values into the formula: x = (-5 ± √(5^2 – 4 * 2 * -3)) / (2 * 2)
- Simplify the expression:
- First, calculate the part within the square root: 5^2 – 4 * 2 * -3 = 29 (because order of operations dictates that squares are done before multiplication).
- Then, find the square root: √29 (Note: This is an irrational number, a number that cannot be expressed as a simple fraction).
- Now, substitute everything back into the formula: x = (-5 ± √29) / (4).
There you have it! The formula provides two possible solutions:
- x = (-5 + √29) / 4 (This is one solution)
- x = (-5 – √29) / 4 (This is the other solution)
Since √29 is an irrational number, these solutions cannot be further simplified into neat fractions or decimals. However, we can express them in this form, which is perfectly acceptable.
Demystifying the Discriminant
The discriminant (b^2 – 4ac) has a significant impact on the solutions we receive from the quadratic formula. Let us see what happens with the discriminant:
- Positive discriminant (b^2 – 4ac > 0): In this case, the discriminant yields two real and unequal solutions. This is what we saw in our example because the square root of 29 provided us with two irrational solutions.
- Zero discriminant (b^2 – 4ac = 0): When the discriminant is zero, the quadratic formula is simplified to x = -b / (2a). Which would indicate simply one repeated real solution. To further understand this type of solution, the graph of the quadratic equation would only touch the x-axis once.
Conclusion
The quadratic formula may seem a tricky equation to understand at first glance, however, it will become an effective tool in your toolbox with practice. Remember, this formula will provide solutions for any quadratic equation, regardless of the complexity of the quadratics.
This blog post has provided you with the fundamentals of quadratic equations, an introduction to the quadratic formula, as well as how to apply it. As you progress through higher secondary school mathematics, you will encounter more difficult quadratic equations. With a solid understanding of this formula, you will be well on your way to unlocking them.
Here are some ways to strengthen your knowledge:
- Practice using the formula with many different quadratic equations. There are many on-line tools and textbooks with practicing problems.
- Explore the relationship between the quadratic formula and the graph of quadratic equations. How are the solutions related to the x-intercepts of the parabola?
- Explore the concept of the discriminant more carefully. How does the determinant affect the characteristics of the solutions both in the case of real numbers, and in the case of negative discriminants?
Remember that mathematics is a process and an exploration. Do not hesitate to play with mathematics, make mistakes and learn from those mistakes. Through hard work and practice you will traverse the quadratic landscape and unlock the answers to these intriguing equations!
Are you looking to inspire your child with math and sharpen their math skills? Moonpreneur’s online math curriculum is different because it teaches your child to understand math skills through effective hands-on lessons, building real-life applications, and engaging in the curiosity to learn math.
You can opt for our Advanced Math or Vedic Math+Mental Math courses. Our Math Quiz for grades 3rd, 4th, 5th, and 6th helps in further exciting and engaging in mathematics with hands-on lessons.
















